Arithmetic Progression (AP)

An arithmetic progression is represented by a,(a + d), (a + 2d), (a + 3d) a + (n – 1)d

Here, a = first term
d = common difference
n = number of terms in the progression

• The general term of an arithmetic progression is given by Tn = a + (n - 1) d.

• The sum of n terms of an arithmetic progression is given by S, = [2a + (n – 1) d] or Sn = 2 [a + l] where l is the last term of arithmetic progression.

• If three numbers are in arithmetic progression, the middle number is called the arithmetic mean of the other two terms.

• If a, b, c are in arithmetic progression, then b = where b is the arithmetic mean.

• Similarly, if ‘n’ terms al, a2, a3… an are in AP, then the arithmetic mean of these ‘n’ terms is given by 

AM =

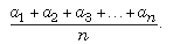
• If the same quantity is added or multiplied to each term of an AP, then the resulting series is also an AP.

• If three terms are in AP, then they can be taken as (a – d), a, (a + d).

• If four terms are in AP, then they can be taken as (a – 3d), (a – d), (a + d), (a + 3d).

• If five terms are in AP, then they can be taken as (a – 2d), (a – d), a, (a + d), (a + 2d).

Geometric Progression (GP)

A geometric progression is represented by a, ar, ar²…ar^n–1.
Here, a = first term
r = common ratio
n = number of terms in the progression.

• The general term of a geometric progression is given by T_n = a^n–1

• The sum to n terms of a geometric progression is given by

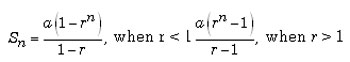
• If three numbers are in geometric progression, the middle number is called the geometric mean of the other two terms.

• If a, b, c are in geometric progression , then 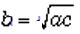where b is the geometric mean.

• Similarly, if n terms a1, a2, a3, a4,…an are in geometric progression, then the geometric mean of 1 these n terms is given by GM = 

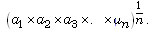
• For a decreasing geometric progression the sum to infinite number of terms is

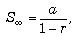where a = first term and | r | < 1.

• If every term of a GP is multiplied by a fixed real number, then the resulting series is also a GP.

• If every term of a GP is raised to the same power, then the resulting series is also a GP.

• The reciprocals of the terms of a GP is also a GP.

Harmonic Progression (HP)

Sum of Natural Series