(Download) SSC: CGL Tier-II Exam Paper-I (Arithmetical Ability) Held On: 16-09-2012

SSC Combined Graduate Level Exam: 2012 (Tier-2)

Paper – I (Held On 16.09.2012)

Quantitative Abilities

1. There are 200 questions on a 3 hour examination. Among 200 questions, 50 are Science. He spent twice, as much time on each Mathematics questions as for each other question. How many minutes did he spend on Mathematics questions?

100
60
36
72

2. The remainder when 9¹⁹+ 6 is divided 8 is

5
7
2
3

3. The least five-digit perfect square number which id divisible by 3, 4, 5, and 8 is

10800
10201
14400
32400

4. Which of the following numbers does not fit into the series?

14, 19, 29, 40, 44, 51, 59, 73

44
29
59
51

5. What will be the remainder when 19¹⁰⁰is divided by 20?

3
1
19
20

6. A toy factory manufactured a batch of electronic toys. If the toys were packed in boxes of 115 each, 13 boxes would not be filled completely. If the boys were packed in boxes of 65 each, 22 such boxes would not be enough to pack all of them. Coincidentally, in the end, the toys were packed n in boxes containing n toys each, without any remainder. The total number of toys was

1444
1454
1424
1434

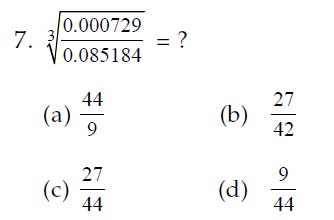
8. Complete the series: 7, 26, 63, 124, 215, 342, __?__

391
421
481
511

9. A woman sells to the first customer half her stock of apples and half an apple, to the second customer half an apple and half of her remaining stock and so also to a third and to a fourth customer. She finds that she has now 15 apples left. How many had she at first?

125
255
250
155

10. A and B can complete a job in 24 days 1 working together. A alone can complete it in 32 days. Both of them worked together for 8 days and them A left. The number of days B will take to complete the remaining job is

64
128
16
32

Click Here To Download PDF

Study Kit for SSC CGL (Tier-2) Exam

DOWNLOAD SSC CGL (Tier-2) Question Papers PDF

DOWNLOAD SSC CGL (Tier-1) Question Papers PDF

DOWNLOAD SSC E-BOOKS PDF

DOWNLOAD SSC CGL Exam Current Affairs PDF

11. In a factory, there are equal number of women and children. Women work for 6 hours a day an children and 4 hours a day. During festival time, the work load goes up by 50%. The government rule does not allow children to work for more than 6 hours a day. If they are equally efficient and the extra work is done by women, then extra hours of work put in by women every day are

4
9
5
3

12. A and B each working alone can do a work in 15 days and 25 days respectively. They started the work together, but B left after some time and A finished the remaining work in 7 days. After how many days from the start did B leave?

7
9
3
5

13. A and B started a business with Rs. 20,000 and Rs. 35,000 respectively. They agreed to share the profit in the ratio of their capital C joins the partnership with the condition and lays Rs. 2,20,000 as premium for this, be shared between A and B. This is to divided between A and B in the ratio of

9 : 10
10 : 9
10 : 1
1 : 10

14. A can complete a work in 20 days and B 30 days. A worked alone for 4 days and the B completed the remaining work along with C in 18 days. In how many days can working alone complete the work?

72
90
12
68

15. A pipe can fill a cistern in 12 minutes and another pipe can fill it in 15 minutes, but third pipe can empty it in 6 minutes. The first two pipes are kept open for 5 minutes in the beginning and then the third pipe also opened. Number of minutes taken empty the cistern is

42
45
38
22

16. Brothers A and B had some savings in the ratio 4 : 5. They decided to buy a gift for their sister, sharing the cost in the ratio 3 : 4. After they bought, A spent two-third of his amount while B is left with Rs. 145. Them the value of the fit is

Rs. 140
Rs. 175
Rs. 70
Rs. 105

17. The taxi charges in a city contain fixed charges and additional charge.km. The fixed charge is for a distance of upto 5 km and additional charge/km thereafter. The charge for a distance of 10 km is Rs. 350 and for 25 km is Rs. 800. The charge for a distance of 30 km is

Rs. 900
Rs. 950
Rs. 800
Rs. 750

18. The marks of 3 students A, B and C are in the ratio 10 : 12 : 15. If the maximum marks of the paper are 100, then the marks of B cannot be in the range of

70 – 80
80 – 90
20 – 30
40 – 50

19. The average of the test scores of a class of ‘m’ students is 70 and that of ‘n’ students is 91. When the scores of both the classes are combined, the average is 80. What is n/m?

10/13
10/11
11/10
13/10

20. The average salary per head of all workers of an institution is Rs. 60. The average salary per head of 12 officers is Rs. 400. The average salary per head of the rest is Rs. 56. Then the total number of workers in the institution is

1062
1060
1030
1032

21. A cricketer played 80 innings and scored an average of 99 runs. His score is in the last inning is zero run. To have an average of 100 at the end, his score in the last innings should have been

60 runs
80 runs
10 runs
1 run

22. A man spends an average of Rs. 1,694.70 per month for the first 7 months and Rs.1,810.50 per month for the next 5 months. His monthly salary if he saves Rs. 3,084.60 during the whole year is

Rs. 1,000
Rs. 2,000
Rs. 2,400
Rs. 3,000

23. A and B undertake to do a piece of work for Rs. 2,200. A alone can do it in 8 days, while B can do it in 6 days. With the help of C, they complete it in 3 days. Find C’s share.

Rs. 150
Rs. 100
Rs. 450
Rs. 300

24. By selling an article at 80% of it marked price, a trader makes a loss of 10%. What will be the profit percentage if he sells it at 95% of its marked price?

5.9
12.5
6.9
5

25. By selling an umbrella for Rs. 30, a shopkeeper gains 20%. During a clearance sale, the shopkeeper allows a discount of 10% of the marked price. He gain during eh sale season is

8
9
7
7.5

26. What is the maximum percentage discount (approximately) that a merchant can offer on his marked price so that he ends up selling at no profit or loss, if he initially marked his goods up by 40%?

33.5%
28.5%
60%
No discount

27. A shopkeeper marks the prices at 15% higher than the original price. Due to increase in demand, he further increase the price by 10%. How much percentage profit will he get?

20
24.5
25
26.5

28. From a vessel containing 100 l of wine, 10 l are drawn out and an equal amount of water is added. From the mixture, 10 l is again drawn out and same quantity of water is added. What is the final ratio of wine and water?

91 : 9
81 : 19
80 : 20
90 : 10

29. From each of two given numbers, half the smaller number is subtracted. After such subtraction, the larger number is 4 times as large as the smaller number. What is the ratio of the numbers?

4 : 1
4 : 5
5 : 2
1 : 4

30. Men, women and children are employed to do a work in the proportion of 3 : 2 : 1 and their wages as 5 : 3 :2. When 90 men are employed, total daily wages of all amounts to Rs. 10,350. Find the daily wage of a man.

Rs. 115
Rs. 75
Rs. 45
Rs. 57.50

31. A sells an article to B making a profit of 1/5 of his outlay. B sells it to C, gaining 20%. If C sells it for Rs. 600 and incurs a loss of 1/6 of his outlay, the cost price of A is

Rs. 720
Rs. 800
Rs. 600
Rs. 500

32. A man had a certain amount with him. He spent 20% of that to buy an article and 5% of the remaining on transport. Then he gifted Rs. 120. If he is left with Rs. 1,400, the amount he spent on transport is

Rs. 95
Rs.80
Rs. 76
Rs. 61

33. The population of a town is 3,11,250. The ratio between women and men is 43 : 40. If there are 24% literate among men and 8% literature among women, the total number of literature persons in the town is

56,800
99,600
41,800
48,900

34. In an examination, 52% of the candidates failed in English and 43% failed in Mathematics. If 17% failed in both the subjects, then the percentage of candidates, who passed in both the subjects, was

25
22
23
21

35. In a election there were only two candidates. One of the candidates secured 40% of votes and is defined by the other candidate by 298 votes. The total number of votes polled is

1490
1500
745
1460

36. P and Q are 27 km away. Two trains with speeds of 24 km/hr and 18 km/hr respectively start simultaneously from P and Q and travel in the same direction. They meet at a point R beyond Q. Distance QR is

48 km
36 km
126 km
81 km

37. A boat covers 12 km upstream in 3 hours, while it covers 36 km upstream and 24 km downstream in hours. What is the speed of the current?

2 km/hr
2.5 km/hr
1.5 km/hr
1 km/hr

38. Average rainfall on Monday, Tuesday, Wednesday and Thursday is 420.5 cm and average on Tuesday, Wednesday, Thursday and Friday is 330.5 cm. If the ratio of rainfall for Monday and Friday is 20 : 21, find the rainfall in cm on Monday and Friday.

1700, 1740
1682, 1762
1800, 1890
1600, 1680

39. The average of 5 consecutive integers starting with ‘m’ is n. What is the average of 6 consecutive integers starting with (m + 2)?

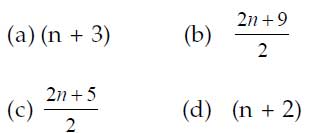
40. A manufacture sells an article to a wholesale dealer at a profit of 10%. The wholesale dealer sells it to a shopkeeper at 20% profit. The shopkeeper sells it to a customer for Rs. 56,100 at a loss of 15%. Then the cost price of the article to the manufacture is

Rs. 50,000
Rs. 55,000
Rs. 25,000
Rs. 10,000

41. A loss of 19% gets converted into a profit on 17% when the selling price in increased by Rs. 162. The cost price of the article is

Rs. 360
Rs. 540
Rs. 450
Rs. 600

42. A man purchased 150 pens at the rate of Rs. 12 per pen. He said 50 pens at a gain of 10%. The percentage gain at which he sell the remaining pens so as to gain 15% of the whole outlay is

43. A dealer sold two types of goods for Rs. 10,000 each. On one of them, he lost 20% and the other he gained 20%. His gain or loss percent in the entire transaction was

4% gain
4% loss
2% loss
20% gain

44. The cost price of 40 articles is the same at the selling price of 25 articles. Find the gain %

15%
75%
65%
60%

45. Water flows at a rate of 10 meters per minute from a cylindrical pipe 5 mm in diameter. How long will it take to fill up a conical vessel whose diameter at the base is 40 cm and depth is 24 cm?

48 mins 15secs
55 mins
51 mins 12 secs
52 mins 1 sec

46. The three perpendicular distances of three sides of an equilateral triangle from a point which lies inside that triangle are 6 cm, 9 cm and 12 cm respectively. The perimeter of the triangle is

55 Ö2 cm
53 Ö3 cm
42Ö2 cm
45 Ö3 cm

47. The area of aright-angled triangle is 34 cm² and one of the sides containing the right angle is 6 cm. The altitude on the hypotenuse is

5.2 cm
12 cm
3.6 cm
4.8 cm

48. A cost of cultivating a square field at a rate of Rs. 135 per hectare is Rs. 1,215. The cost of putting a fence around it at the rate of 75 paise per metre would be

Rs. 900
Rs. 1,800
Rs. 360
Rs. 810

49. The area of a trapezium is 384 cm². IF its parallel sides are in the ratio 3 : 5 and the perpendicular distance between them is 12 cm, the smaller of the parallel sides is

30 cm
36 cm
20 cm
24 cm

50. The perimeter of the triangular base of a right prism is 60 cm and the sides of the base are in the ratio 5 : 12 : 13. Then its volume will be (height of the prism being 50 cm)

5400 cm³
9600 cm³
6000 cm⁴
6600 cm³

51. If the length of a rectangular parallelepiped is 3 times of its breadth and 5 times of its height and its volume is 144400 cu.cm, then area of the total surface will be

4320 sq. cm
5320 sq. cm
2420 sq. cm
3320 sq. cm

52. The capacities of two hemispherical bows are 6.4 litres and 21.6 litres respectively. Then the ratio of their internal curved surface areas will be

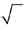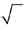2 : 3
16 : 31
4 : 9
2 : 3

53. A man can row at 10 kmph in still water. If it takes a total of 5 hours four him to go to a place 24 km away and return, then the speed of the water current is

(a) 1/2 kmph

(b) 1 kmph

(c) 2 kmph

(d) 3 kmph

54. A man started 20 minutes late and travelling at a speed of 3/2 times of his usual speed reaches his office in time. The time taken by the man to reach his office at his usual speed is

1 hour
30 minutes
40 minutes
1 hr 20 minutes

55. Divide Rs. 15,494 A and B so that A’s share at the end of 9 years may be equal to B’s share at the end of 11 years, compound interest being 20% per annum. Then A’s share is

Rs. 9144
Rs. 9414
Rs. 8000
Rs. 9140

56. The principal amount which yields a compound interest or Rs. 208 in the second year at 4% is

Rs. 13000
Rs. 6500
Rs. 5000
Rs. 10000

57. An amount is invested in a bank at compound rate of interest, after first and third year is Rs. 1200 and Rs. 1587 respectively. What is the rate of interest?

12%
15%
10%
3.9%

58. The difference between compound and simple rates of interest on Rs. 10000 for 3 years at 5% p.a. is

Rs. 76.50
Rs. 76
Rs. 76.25
Rs. 76.75

59. A solid consists of a circular cylinder with exact fitting right circular cone placed on the top. The height of the cone is h. If total volume of the solid is three times the volume of the cone, then the height of the circular cylinder is

4 h
3h/2
2h
2h/3

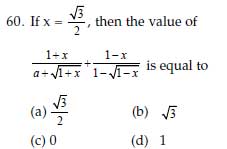
61. The area of the region bounded by y = |x| - 5 with the co-ordinate axes is

50 sq. units
20 sq. units
25 sq. units
52 sq. units

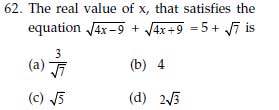
63. Find the value of a³ + b³ + c³ – 3abc when a = 225, b = 236, c = 227.

2034
2340
2304
2430

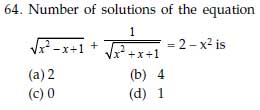
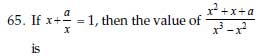
2/a
-2/a
-2
-a/2

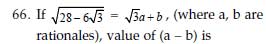
1
-1
-2
2

67. 2³² – (2 + 1) (2² – 1) (2⁴ + 1) (2⁸ + 1) (2¹⁶ + 1) is equal to

2
2¹⁶
0
1

68. If the expression x + 809436 × 809438 be a perfect square, then the value of x is

809436
809438
0
1

69. If O is the orthocenter of the AND = 80^O, then measure of is

120^o
90^o
80^o
100^o

70. Let A and B be two solid spheres such that the surface area of B is 300% higher than the surface area of A. The volume of A is found to be k% lower than the volume of B. The value of k must be

90.5
87.5
85.5
92.5

71. The ratio of the areas of the circumcircle and the in circle of a square is

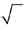2 : 1
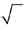1 : 2
2 : 1
1 : 2

72. From a circular sheet of paper of radius 10 cm, a sector of area 40% is removed. If the remaining part is used to make a conical surface, then the ratio of the radius and the height of the cone is

3 : 4
4 : 3
1 : 2
1 : 1

73. If the area of the circular shell having inner and outer radii of 8 cm and 12 cm respectively is equal to the total surface area of a cylinder of radius R₁ and height h, then h, is terms of R₁ will be

74. A well or radius 3.5 m is dug 16 m deep. The earth removed is spread over an area of 400 m² to form a platform. Height of the platform is

7.7 m
77 m
1.57 m
154 m

75. The ratio of the number of sides of two regular polygons is 1 : 2. If each interior angle of the first polygon is 120^o, then the measure of each interior angle of the second polygon is

150^o
160^o
140^o
135^o

2
5
0
1

77. Two posts are x meters apart and the height of one is double that of the other. If from the mid-point of the line joining their feet, an observer finds the angular elevations of their tops to be complementary, then the height (in meters) of the shorter post is

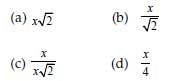
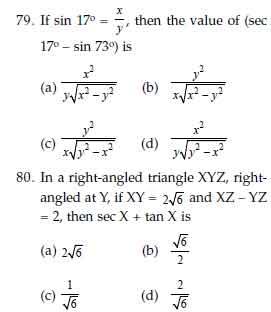
81. If 0^o < < 90^o, then the value of sin + cos is

less than 1
equal to 2
equal to 1
greater than 1

82. An aeroplane when flying at a height of 5000 m from the ground passes vertically above another aeroplane at an instant, when the angles of elevation of the two aeroplanes from the same point on the ground are 60^o and 45^o respectively. The vertical distance between the aeroplanes at the instant is

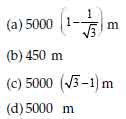
83. The angles of a triangle are in Arithmetic Progression. The ratio of the latest angle in degrees to the number of radians in the greatest angle is 60 : . The angles in degrees are

40^o, 50^o, 90^o
40^o, 55^o, 85^o
30^o, 60^o, 90^o
35^o, 55^o, 90^o

84. Suppose be a right-angled triangle where = 90^o, and AD BC. If = 40cm², = 10 cm² and AC = 9 cm, then the length of BC is

4 cm
6 cm
12 cm
8 cm

85. Two circles touch each other externally at P. AB is a direct common tangent to the two circles, A and B are points of contact and = 35^o. Then is

65^o
75^o
35^o
55^o

86. The length of the common chord of two interesting circles is 24 cm. If the diameters of the circles are 30 cm and 26 cm, then the distance between the centres in cm is

15
16
13
14

87. In, D and E are points on AB and AC respectively such that DE | BC and DE divides the into two parts of equal areas. Then ratio of AD and BD is

1 : 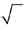2
1 : 2+1
1 : 1
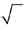1 : 2 - 1

88. The area of the square inscribed in a circle of radius 8 cm is

128 sq. cm
125 sq. cm
256 sq. cm
250 sq. cm

89. X and Y are centres of circles of radii 9 cm and 2 cm respectively, XY = 17 cm. Z is the centre of a circle of radius r cm which touches the above circles externally. Given that = 90^o, the value of r is

9 cm
8 cm
13 cm
6 cm

90. I is the in centre of a triangle ABC. If = 65^o and = 55^o, then the value of is

140^o
110^o
130^o
120^o

91. If the radii of two circles be 6 cm and 3 cm and the length of the transverse common tangent be 8 cm, then the distance between the two centres is

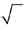150 cm
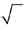135 cm
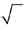145 cm
140 cm

92. The ratio between the number of sides of two regular polygons is 1 : 2 and the ratio between their interior angles is 2 : 3. The number of sides of these polygons is respectively

4, 8
7, 14
6, 12
5, 10

93. The minimum value of Sin²+ cos²+ sec² + cosec² + tan² + cot²is

5
7
1
3

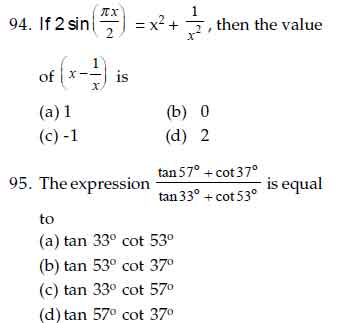
Directions: The bar chart given below shows the percentage distribution of the production of various models of a mobile manufacturing company in 2007 and 2008. The total production in 2007 was 35 lakh mobile phones and in 2008 the production was 44 lakh. Study the chart and answer questions 96 – 100.

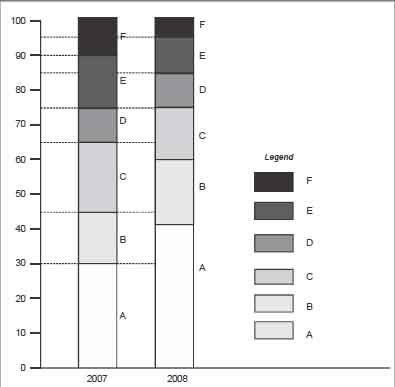
96. What was the difference in the number of B type mobile produced in 2007 and 2008?

2,25,000
1,75,000
3,55,000
2,70,000

97. If the percentage production of A type mobiles in 2008 was same as that in 2007, then the number of A type mobile produced in 2008 would have been